Input interpretation
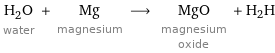
H_2O water + Mg magnesium ⟶ MgO magnesium oxide + H2H
Balanced equation

Balance the chemical equation algebraically: H_2O + Mg ⟶ MgO + H2H Add stoichiometric coefficients, c_i, to the reactants and products: c_1 H_2O + c_2 Mg ⟶ c_3 MgO + c_4 H2H Set the number of atoms in the reactants equal to the number of atoms in the products for H, O and Mg: H: | 2 c_1 = 3 c_4 O: | c_1 = c_3 Mg: | c_2 = c_3 Since the coefficients are relative quantities and underdetermined, choose a coefficient to set arbitrarily. To keep the coefficients small, the arbitrary value is ordinarily one. For instance, set c_4 = 1 and solve the system of equations for the remaining coefficients: c_1 = 3/2 c_2 = 3/2 c_3 = 3/2 c_4 = 1 Multiply by the least common denominator, 2, to eliminate fractional coefficients: c_1 = 3 c_2 = 3 c_3 = 3 c_4 = 2 Substitute the coefficients into the chemical reaction to obtain the balanced equation: Answer: | | 3 H_2O + 3 Mg ⟶ 3 MgO + 2 H2H
Structures
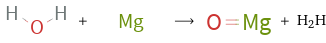
+ ⟶ + H2H
Names
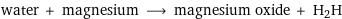
water + magnesium ⟶ magnesium oxide + H2H
Equilibrium constant
![Construct the equilibrium constant, K, expression for: H_2O + Mg ⟶ MgO + H2H Plan: • Balance the chemical equation. • Determine the stoichiometric numbers. • Assemble the activity expression for each chemical species. • Use the activity expressions to build the equilibrium constant expression. Write the balanced chemical equation: 3 H_2O + 3 Mg ⟶ 3 MgO + 2 H2H Assign stoichiometric numbers, ν_i, using the stoichiometric coefficients, c_i, from the balanced chemical equation in the following manner: ν_i = -c_i for reactants and ν_i = c_i for products: chemical species | c_i | ν_i H_2O | 3 | -3 Mg | 3 | -3 MgO | 3 | 3 H2H | 2 | 2 Assemble the activity expressions accounting for the state of matter and ν_i: chemical species | c_i | ν_i | activity expression H_2O | 3 | -3 | ([H2O])^(-3) Mg | 3 | -3 | ([Mg])^(-3) MgO | 3 | 3 | ([MgO])^3 H2H | 2 | 2 | ([H2H])^2 The equilibrium constant symbol in the concentration basis is: K_c Mulitply the activity expressions to arrive at the K_c expression: Answer: | | K_c = ([H2O])^(-3) ([Mg])^(-3) ([MgO])^3 ([H2H])^2 = (([MgO])^3 ([H2H])^2)/(([H2O])^3 ([Mg])^3)](../image_source/7211285a00d0019b1089d062d46cba76.png)
Construct the equilibrium constant, K, expression for: H_2O + Mg ⟶ MgO + H2H Plan: • Balance the chemical equation. • Determine the stoichiometric numbers. • Assemble the activity expression for each chemical species. • Use the activity expressions to build the equilibrium constant expression. Write the balanced chemical equation: 3 H_2O + 3 Mg ⟶ 3 MgO + 2 H2H Assign stoichiometric numbers, ν_i, using the stoichiometric coefficients, c_i, from the balanced chemical equation in the following manner: ν_i = -c_i for reactants and ν_i = c_i for products: chemical species | c_i | ν_i H_2O | 3 | -3 Mg | 3 | -3 MgO | 3 | 3 H2H | 2 | 2 Assemble the activity expressions accounting for the state of matter and ν_i: chemical species | c_i | ν_i | activity expression H_2O | 3 | -3 | ([H2O])^(-3) Mg | 3 | -3 | ([Mg])^(-3) MgO | 3 | 3 | ([MgO])^3 H2H | 2 | 2 | ([H2H])^2 The equilibrium constant symbol in the concentration basis is: K_c Mulitply the activity expressions to arrive at the K_c expression: Answer: | | K_c = ([H2O])^(-3) ([Mg])^(-3) ([MgO])^3 ([H2H])^2 = (([MgO])^3 ([H2H])^2)/(([H2O])^3 ([Mg])^3)
Rate of reaction
![Construct the rate of reaction expression for: H_2O + Mg ⟶ MgO + H2H Plan: • Balance the chemical equation. • Determine the stoichiometric numbers. • Assemble the rate term for each chemical species. • Write the rate of reaction expression. Write the balanced chemical equation: 3 H_2O + 3 Mg ⟶ 3 MgO + 2 H2H Assign stoichiometric numbers, ν_i, using the stoichiometric coefficients, c_i, from the balanced chemical equation in the following manner: ν_i = -c_i for reactants and ν_i = c_i for products: chemical species | c_i | ν_i H_2O | 3 | -3 Mg | 3 | -3 MgO | 3 | 3 H2H | 2 | 2 The rate term for each chemical species, B_i, is 1/ν_i(Δ[B_i])/(Δt) where [B_i] is the amount concentration and t is time: chemical species | c_i | ν_i | rate term H_2O | 3 | -3 | -1/3 (Δ[H2O])/(Δt) Mg | 3 | -3 | -1/3 (Δ[Mg])/(Δt) MgO | 3 | 3 | 1/3 (Δ[MgO])/(Δt) H2H | 2 | 2 | 1/2 (Δ[H2H])/(Δt) (for infinitesimal rate of change, replace Δ with d) Set the rate terms equal to each other to arrive at the rate expression: Answer: | | rate = -1/3 (Δ[H2O])/(Δt) = -1/3 (Δ[Mg])/(Δt) = 1/3 (Δ[MgO])/(Δt) = 1/2 (Δ[H2H])/(Δt) (assuming constant volume and no accumulation of intermediates or side products)](../image_source/e02a1bb34bb65043cacad32882aa0d00.png)
Construct the rate of reaction expression for: H_2O + Mg ⟶ MgO + H2H Plan: • Balance the chemical equation. • Determine the stoichiometric numbers. • Assemble the rate term for each chemical species. • Write the rate of reaction expression. Write the balanced chemical equation: 3 H_2O + 3 Mg ⟶ 3 MgO + 2 H2H Assign stoichiometric numbers, ν_i, using the stoichiometric coefficients, c_i, from the balanced chemical equation in the following manner: ν_i = -c_i for reactants and ν_i = c_i for products: chemical species | c_i | ν_i H_2O | 3 | -3 Mg | 3 | -3 MgO | 3 | 3 H2H | 2 | 2 The rate term for each chemical species, B_i, is 1/ν_i(Δ[B_i])/(Δt) where [B_i] is the amount concentration and t is time: chemical species | c_i | ν_i | rate term H_2O | 3 | -3 | -1/3 (Δ[H2O])/(Δt) Mg | 3 | -3 | -1/3 (Δ[Mg])/(Δt) MgO | 3 | 3 | 1/3 (Δ[MgO])/(Δt) H2H | 2 | 2 | 1/2 (Δ[H2H])/(Δt) (for infinitesimal rate of change, replace Δ with d) Set the rate terms equal to each other to arrive at the rate expression: Answer: | | rate = -1/3 (Δ[H2O])/(Δt) = -1/3 (Δ[Mg])/(Δt) = 1/3 (Δ[MgO])/(Δt) = 1/2 (Δ[H2H])/(Δt) (assuming constant volume and no accumulation of intermediates or side products)
Chemical names and formulas
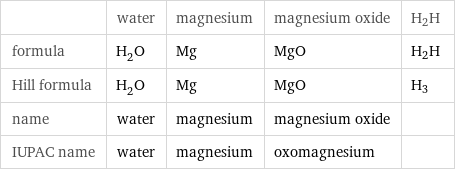
| water | magnesium | magnesium oxide | H2H formula | H_2O | Mg | MgO | H2H Hill formula | H_2O | Mg | MgO | H3 name | water | magnesium | magnesium oxide | IUPAC name | water | magnesium | oxomagnesium |
Substance properties
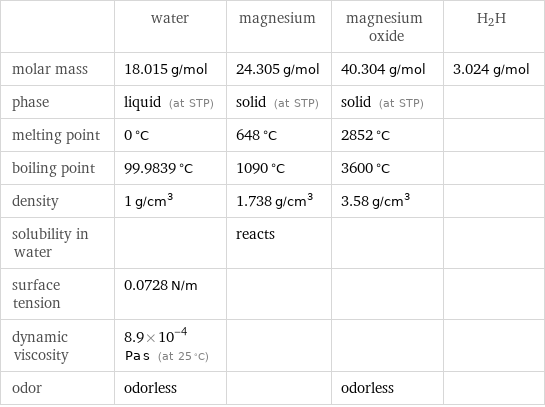
| water | magnesium | magnesium oxide | H2H molar mass | 18.015 g/mol | 24.305 g/mol | 40.304 g/mol | 3.024 g/mol phase | liquid (at STP) | solid (at STP) | solid (at STP) | melting point | 0 °C | 648 °C | 2852 °C | boiling point | 99.9839 °C | 1090 °C | 3600 °C | density | 1 g/cm^3 | 1.738 g/cm^3 | 3.58 g/cm^3 | solubility in water | | reacts | | surface tension | 0.0728 N/m | | | dynamic viscosity | 8.9×10^-4 Pa s (at 25 °C) | | | odor | odorless | | odorless |
Units
