Input interpretation

pKa
Equation

pK_a = -log_10(K_a) | K_a | acidity constant pK_a | log acidity constant (assuming concentrations are in moles/liter)
Input value

log acidity constant | 3.85
Results

acidity constant | 1.413×10^-4
Possible intermediate steps
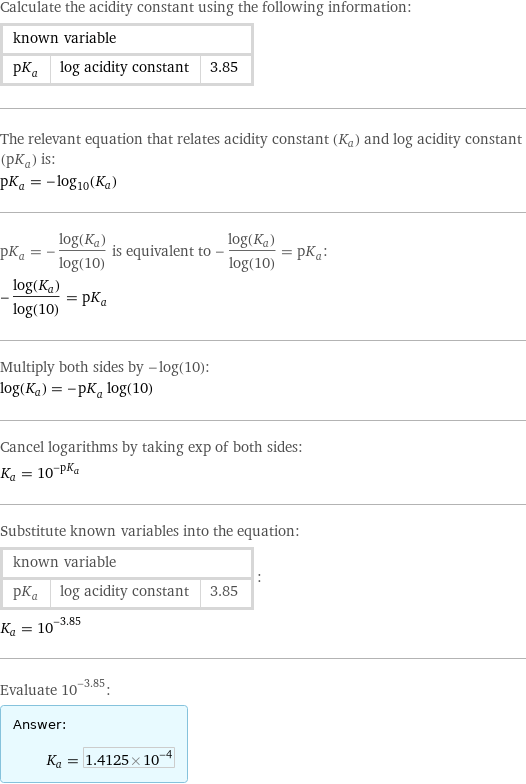
Calculate the acidity constant using the following information: known variable | | pK_a | log acidity constant | 3.85 The relevant equation that relates acidity constant (K_a) and log acidity constant (pK_a) is: pK_a = -log_10(K_a) pK_a = -(log(K_a))/log(10) is equivalent to -(log(K_a))/log(10) = pK_a: -(log(K_a))/log(10) = pK_a Multiply both sides by -log(10): log(K_a) = -pK_a log(10) Cancel logarithms by taking exp of both sides: K_a = 10^(-pK_a) Substitute known variables into the equation: known variable | | pK_a | log acidity constant | 3.85 | : K_a = 10^(-3.85) Evaluate 10^(-3.85): Answer: | | K_a = 1.4125×10^-4