Input interpretation
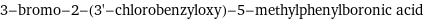
3-bromo-2-(3'-chlorobenzyloxy)-5-methylphenylboronic acid
Basic properties

molar mass | 355.4 g/mol formula | C_14H_13BBrClO_3 empirical formula | Br_C_14B_O_3Cl_H_13 SMILES identifier | CC1=CC(=C(C(=C1)Br)OCC2=CC(=CC=C2)Cl)B(O)O InChI identifier | InChI=1/C14H13BBrClO3/c1-9-5-12(15(18)19)14(13(16)6-9)20-8-10-3-2-4-11(17)7-10/h2-7, 18-19H, 8H2, 1H3 InChI key | GKYZLQGDWVHPPQ-UHFFFAOYSA-N
Lewis structure
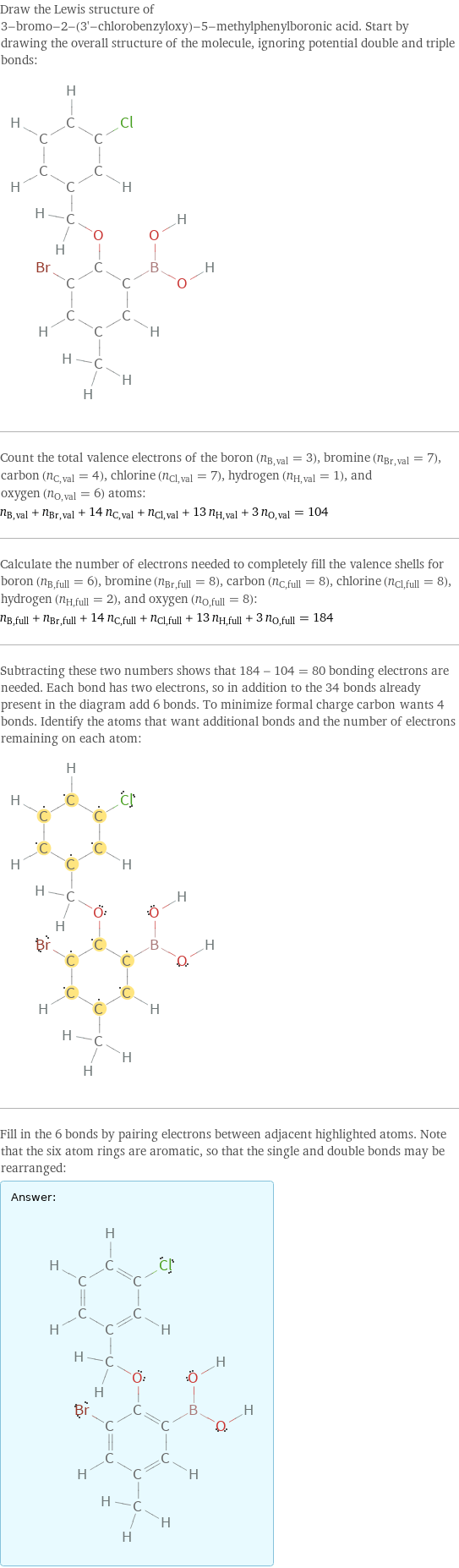
Draw the Lewis structure of 3-bromo-2-(3'-chlorobenzyloxy)-5-methylphenylboronic acid. Start by drawing the overall structure of the molecule, ignoring potential double and triple bonds: Count the total valence electrons of the boron (n_B, val = 3), bromine (n_Br, val = 7), carbon (n_C, val = 4), chlorine (n_Cl, val = 7), hydrogen (n_H, val = 1), and oxygen (n_O, val = 6) atoms: n_B, val + n_Br, val + 14 n_C, val + n_Cl, val + 13 n_H, val + 3 n_O, val = 104 Calculate the number of electrons needed to completely fill the valence shells for boron (n_B, full = 6), bromine (n_Br, full = 8), carbon (n_C, full = 8), chlorine (n_Cl, full = 8), hydrogen (n_H, full = 2), and oxygen (n_O, full = 8): n_B, full + n_Br, full + 14 n_C, full + n_Cl, full + 13 n_H, full + 3 n_O, full = 184 Subtracting these two numbers shows that 184 - 104 = 80 bonding electrons are needed. Each bond has two electrons, so in addition to the 34 bonds already present in the diagram add 6 bonds. To minimize formal charge carbon wants 4 bonds. Identify the atoms that want additional bonds and the number of electrons remaining on each atom: Fill in the 6 bonds by pairing electrons between adjacent highlighted atoms. Note that the six atom rings are aromatic, so that the single and double bonds may be rearranged: Answer: | |
Quantitative molecular descriptors

longest chain length | 11 atoms longest straight chain length | 3 atoms longest aliphatic chain length | 0 atoms aromatic atom count | 12 atoms H-bond acceptor count | 2 atoms H-bond donor count | 2 atoms
Elemental composition
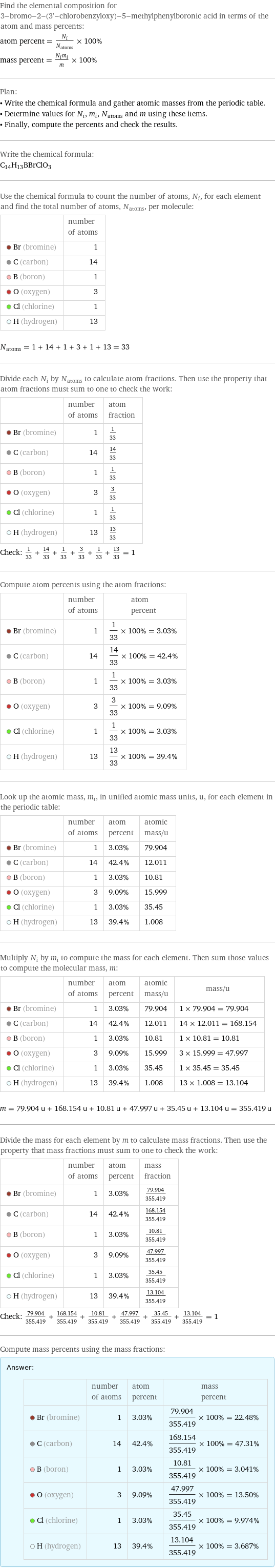
Find the elemental composition for 3-bromo-2-(3'-chlorobenzyloxy)-5-methylphenylboronic acid in terms of the atom and mass percents: atom percent = N_i/N_atoms × 100% mass percent = (N_im_i)/m × 100% Plan: • Write the chemical formula and gather atomic masses from the periodic table. • Determine values for N_i, m_i, N_atoms and m using these items. • Finally, compute the percents and check the results. Write the chemical formula: C_14H_13BBrClO_3 Use the chemical formula to count the number of atoms, N_i, for each element and find the total number of atoms, N_atoms, per molecule: | number of atoms Br (bromine) | 1 C (carbon) | 14 B (boron) | 1 O (oxygen) | 3 Cl (chlorine) | 1 H (hydrogen) | 13 N_atoms = 1 + 14 + 1 + 3 + 1 + 13 = 33 Divide each N_i by N_atoms to calculate atom fractions. Then use the property that atom fractions must sum to one to check the work: | number of atoms | atom fraction Br (bromine) | 1 | 1/33 C (carbon) | 14 | 14/33 B (boron) | 1 | 1/33 O (oxygen) | 3 | 3/33 Cl (chlorine) | 1 | 1/33 H (hydrogen) | 13 | 13/33 Check: 1/33 + 14/33 + 1/33 + 3/33 + 1/33 + 13/33 = 1 Compute atom percents using the atom fractions: | number of atoms | atom percent Br (bromine) | 1 | 1/33 × 100% = 3.03% C (carbon) | 14 | 14/33 × 100% = 42.4% B (boron) | 1 | 1/33 × 100% = 3.03% O (oxygen) | 3 | 3/33 × 100% = 9.09% Cl (chlorine) | 1 | 1/33 × 100% = 3.03% H (hydrogen) | 13 | 13/33 × 100% = 39.4% Look up the atomic mass, m_i, in unified atomic mass units, u, for each element in the periodic table: | number of atoms | atom percent | atomic mass/u Br (bromine) | 1 | 3.03% | 79.904 C (carbon) | 14 | 42.4% | 12.011 B (boron) | 1 | 3.03% | 10.81 O (oxygen) | 3 | 9.09% | 15.999 Cl (chlorine) | 1 | 3.03% | 35.45 H (hydrogen) | 13 | 39.4% | 1.008 Multiply N_i by m_i to compute the mass for each element. Then sum those values to compute the molecular mass, m: | number of atoms | atom percent | atomic mass/u | mass/u Br (bromine) | 1 | 3.03% | 79.904 | 1 × 79.904 = 79.904 C (carbon) | 14 | 42.4% | 12.011 | 14 × 12.011 = 168.154 B (boron) | 1 | 3.03% | 10.81 | 1 × 10.81 = 10.81 O (oxygen) | 3 | 9.09% | 15.999 | 3 × 15.999 = 47.997 Cl (chlorine) | 1 | 3.03% | 35.45 | 1 × 35.45 = 35.45 H (hydrogen) | 13 | 39.4% | 1.008 | 13 × 1.008 = 13.104 m = 79.904 u + 168.154 u + 10.81 u + 47.997 u + 35.45 u + 13.104 u = 355.419 u Divide the mass for each element by m to calculate mass fractions. Then use the property that mass fractions must sum to one to check the work: | number of atoms | atom percent | mass fraction Br (bromine) | 1 | 3.03% | 79.904/355.419 C (carbon) | 14 | 42.4% | 168.154/355.419 B (boron) | 1 | 3.03% | 10.81/355.419 O (oxygen) | 3 | 9.09% | 47.997/355.419 Cl (chlorine) | 1 | 3.03% | 35.45/355.419 H (hydrogen) | 13 | 39.4% | 13.104/355.419 Check: 79.904/355.419 + 168.154/355.419 + 10.81/355.419 + 47.997/355.419 + 35.45/355.419 + 13.104/355.419 = 1 Compute mass percents using the mass fractions: Answer: | | | number of atoms | atom percent | mass percent Br (bromine) | 1 | 3.03% | 79.904/355.419 × 100% = 22.48% C (carbon) | 14 | 42.4% | 168.154/355.419 × 100% = 47.31% B (boron) | 1 | 3.03% | 10.81/355.419 × 100% = 3.041% O (oxygen) | 3 | 9.09% | 47.997/355.419 × 100% = 13.50% Cl (chlorine) | 1 | 3.03% | 35.45/355.419 × 100% = 9.974% H (hydrogen) | 13 | 39.4% | 13.104/355.419 × 100% = 3.687%
Elemental oxidation states
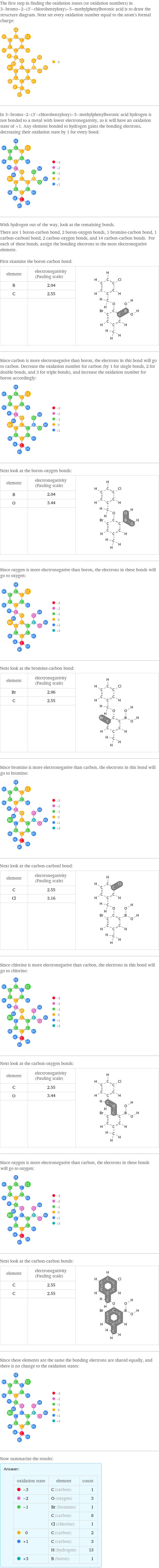
The first step in finding the oxidation states (or oxidation numbers) in 3-bromo-2-(3'-chlorobenzyloxy)-5-methylphenylboronic acid is to draw the structure diagram. Next set every oxidation number equal to the atom's formal charge: In 3-bromo-2-(3'-chlorobenzyloxy)-5-methylphenylboronic acid hydrogen is not bonded to a metal with lower electronegativity, so it will have an oxidation state of +1. Any element bonded to hydrogen gains the bonding electrons, decreasing their oxidation state by 1 for every bond: With hydrogen out of the way, look at the remaining bonds. There are 1 boron-carbon bond, 2 boron-oxygen bonds, 1 bromine-carbon bond, 1 carbon-carbonl bond, 2 carbon-oxygen bonds, and 14 carbon-carbon bonds. For each of these bonds, assign the bonding electrons to the most electronegative element. First examine the boron-carbon bond: element | electronegativity (Pauling scale) | B | 2.04 | C | 2.55 | | | Since carbon is more electronegative than boron, the electrons in this bond will go to carbon. Decrease the oxidation number for carbon (by 1 for single bonds, 2 for double bonds, and 3 for triple bonds), and increase the oxidation number for boron accordingly: Next look at the boron-oxygen bonds: element | electronegativity (Pauling scale) | B | 2.04 | O | 3.44 | | | Since oxygen is more electronegative than boron, the electrons in these bonds will go to oxygen: Next look at the bromine-carbon bond: element | electronegativity (Pauling scale) | Br | 2.96 | C | 2.55 | | | Since bromine is more electronegative than carbon, the electrons in this bond will go to bromine: Next look at the carbon-carbonl bond: element | electronegativity (Pauling scale) | C | 2.55 | Cl | 3.16 | | | Since chlorine is more electronegative than carbon, the electrons in this bond will go to chlorine: Next look at the carbon-oxygen bonds: element | electronegativity (Pauling scale) | C | 2.55 | O | 3.44 | | | Since oxygen is more electronegative than carbon, the electrons in these bonds will go to oxygen: Next look at the carbon-carbon bonds: element | electronegativity (Pauling scale) | C | 2.55 | C | 2.55 | | | Since these elements are the same the bonding electrons are shared equally, and there is no change to the oxidation states: Now summarize the results: Answer: | | oxidation state | element | count -3 | C (carbon) | 1 -2 | O (oxygen) | 3 -1 | Br (bromine) | 1 | C (carbon) | 8 | Cl (chlorine) | 1 0 | C (carbon) | 2 +1 | C (carbon) | 3 | H (hydrogen) | 13 +3 | B (boron) | 1
Orbital hybridization
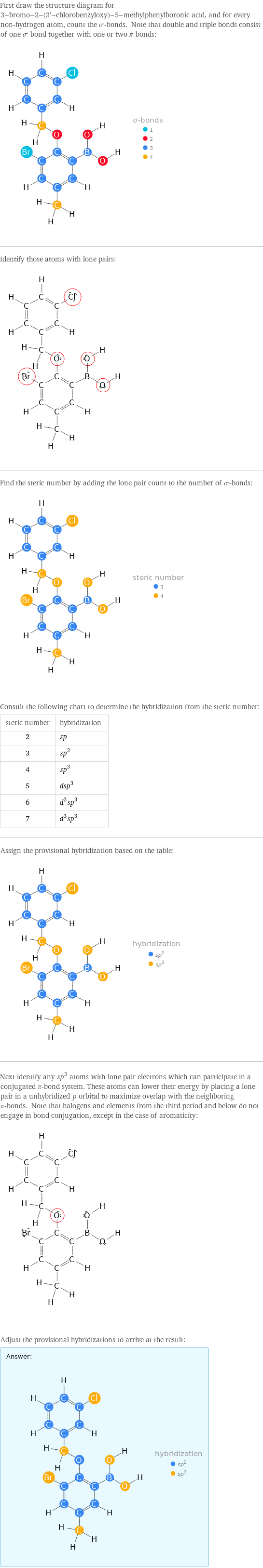
First draw the structure diagram for 3-bromo-2-(3'-chlorobenzyloxy)-5-methylphenylboronic acid, and for every non-hydrogen atom, count the σ-bonds. Note that double and triple bonds consist of one σ-bond together with one or two π-bonds: Identify those atoms with lone pairs: Find the steric number by adding the lone pair count to the number of σ-bonds: Consult the following chart to determine the hybridization from the steric number: steric number | hybridization 2 | sp 3 | sp^2 4 | sp^3 5 | dsp^3 6 | d^2sp^3 7 | d^3sp^3 Assign the provisional hybridization based on the table: Next identify any sp^3 atoms with lone pair electrons which can participate in a conjugated π-bond system. These atoms can lower their energy by placing a lone pair in a unhybridized p orbital to maximize overlap with the neighboring π-bonds. Note that halogens and elements from the third period and below do not engage in bond conjugation, except in the case of aromaticity: Adjust the provisional hybridizations to arrive at the result: Answer: | |
Topological indices
vertex count | 33 edge count | 34 Schultz index | 11122 Wiener index | 2816 Hosoya index | 2.06×10^6 Balaban index | 2.465