Input interpretation

silver (mineral) | crystal system | tetraferroplatinum (mineral) | crystal system
Result

cubic | tetragonal
Basic properties
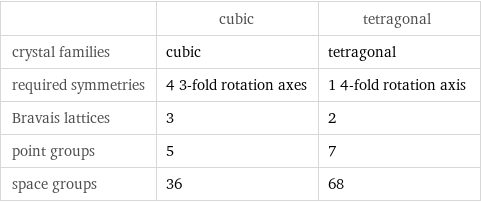
| cubic | tetragonal crystal families | cubic | tetragonal required symmetries | 4 3-fold rotation axes | 1 4-fold rotation axis Bravais lattices | 3 | 2 point groups | 5 | 7 space groups | 36 | 68
Lattice properties
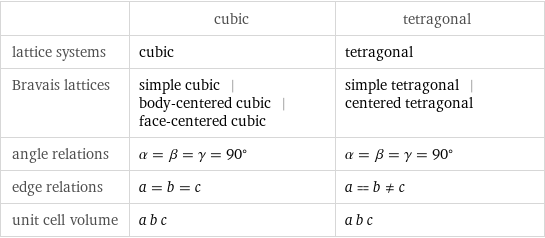
| cubic | tetragonal lattice systems | cubic | tetragonal Bravais lattices | simple cubic | body-centered cubic | face-centered cubic | simple tetragonal | centered tetragonal angle relations | α = β = γ = 90° | α = β = γ = 90° edge relations | a = b = c | a = b!=c unit cell volume | a b c | a b c
Corresponding symmetry groups
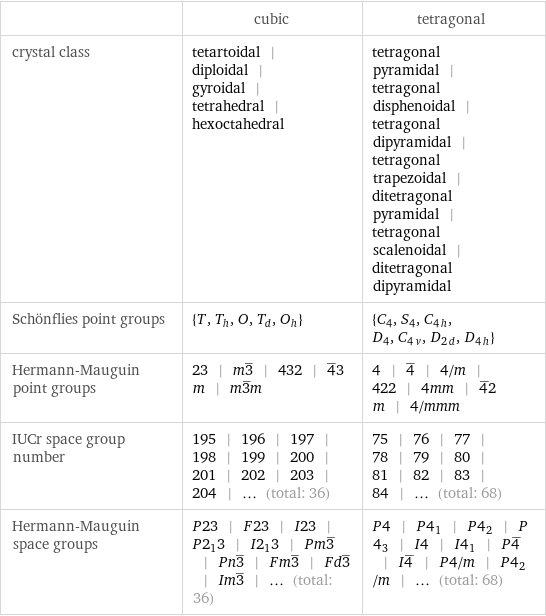
| cubic | tetragonal crystal class | tetartoidal | diploidal | gyroidal | tetrahedral | hexoctahedral | tetragonal pyramidal | tetragonal disphenoidal | tetragonal dipyramidal | tetragonal trapezoidal | ditetragonal pyramidal | tetragonal scalenoidal | ditetragonal dipyramidal Schönflies point groups | {T, T_h, O, T_d, O_h} | {C_4, S_4, C_4h, D_4, C_4v, D_2d, D_4h} Hermann-Mauguin point groups | 23 | m3^_ | 432 | 4^_3m | m3^_m | 4 | 4^_ | 4/m | 422 | 4mm | 4^_2m | 4/mmm IUCr space group number | 195 | 196 | 197 | 198 | 199 | 200 | 201 | 202 | 203 | 204 | ... (total: 36) | 75 | 76 | 77 | 78 | 79 | 80 | 81 | 82 | 83 | 84 | ... (total: 68) Hermann-Mauguin space groups | P23 | F23 | I23 | P2_13 | I2_13 | Pm3^_ | Pn3^_ | Fm3^_ | Fd3^_ | Im3^_ | ... (total: 36) | P4 | P4_1 | P4_2 | P4_3 | I4 | I4_1 | P4^_ | I4^_ | P4/m | P4_2/m | ... (total: 68)