Input interpretation
monoprotic strong acid-strong base titration
Equation
![V_a [H^+] = V_b [OH^-] | V_a | volume of acid V_b | volume of base [OH^-] | base concentration [H^+] | acid concentration (assuming equivalence point of monoprotic acid & base)](../image_source/0be61ededc421dbc1281a04feb966aa4.png)
V_a [H^+] = V_b [OH^-] | V_a | volume of acid V_b | volume of base [OH^-] | base concentration [H^+] | acid concentration (assuming equivalence point of monoprotic acid & base)
Input values
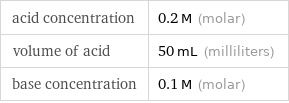
acid concentration | 0.2 M (molar) volume of acid | 50 mL (milliliters) base concentration | 0.1 M (molar)
Results

volume of base | 100 mL (milliliters) = 100 cm^3 (cubic centimeters) = 1×10^-4 m^3 (cubic meters) = 0.02642 gallons = 1 dL (deciliter) = 0.1 L (liters)
Possible intermediate steps
![Calculate the volume of base using the following information: known variables | | [H^+] | acid concentration | 0.2 M V_a | volume of acid | 50 mL [OH^-] | base concentration | 0.1 M Convert known variables into appropriate units using the following: 1 M = 1000 mol/m^3: 1 mL = 1×10^-6 m^3: 1 M = 1000 mol/m^3: known variables | | [H^+] | acid concentration | 200 mol/m^3 V_a | volume of acid | 5×10^-5 m^3 [OH^-] | base concentration | 100 mol/m^3 The relevant equation that relates volume of base (V_b), acid concentration ([H^+]), volume of acid (V_a), and base concentration ([OH^-]) is: V_a [H^+] = V_b [OH^-] V_a [H^+] = V_b [OH^-] is equivalent to V_b [OH^-] = V_a [H^+]: V_b [OH^-] = V_a [H^+] Divide both sides by [OH^-]: V_b = (V_a [H^+])/([OH^-]) Substitute known variables into the equation: known variables | | [H^+] | acid concentration | 200 mol/m^3 V_a | volume of acid | 5×10^-5 m^3 [OH^-] | base concentration | 100 mol/m^3 | : V_b = (200 mol/m^3×5×10^-5 m^3)/(100 mol/m^3) Separate the numerical part, (200×5×10^-5)/100, from the unit part, (mol/m^3 m^3)/(mol/m^3) = m^3: V_b = (200×5×10^-5)/100 m^3 Evaluate (200×5×10^-5)/100: V_b = 1×10^-4 m^3 Convert 1×10^-4 m^3 into mL (milliliters) using the following: 1 m^3 = 1×10^6 mL: Answer: | | V_b = 100 mL](../image_source/cca7f298f3c228b2241d6ab36fa8bd96.png)
Calculate the volume of base using the following information: known variables | | [H^+] | acid concentration | 0.2 M V_a | volume of acid | 50 mL [OH^-] | base concentration | 0.1 M Convert known variables into appropriate units using the following: 1 M = 1000 mol/m^3: 1 mL = 1×10^-6 m^3: 1 M = 1000 mol/m^3: known variables | | [H^+] | acid concentration | 200 mol/m^3 V_a | volume of acid | 5×10^-5 m^3 [OH^-] | base concentration | 100 mol/m^3 The relevant equation that relates volume of base (V_b), acid concentration ([H^+]), volume of acid (V_a), and base concentration ([OH^-]) is: V_a [H^+] = V_b [OH^-] V_a [H^+] = V_b [OH^-] is equivalent to V_b [OH^-] = V_a [H^+]: V_b [OH^-] = V_a [H^+] Divide both sides by [OH^-]: V_b = (V_a [H^+])/([OH^-]) Substitute known variables into the equation: known variables | | [H^+] | acid concentration | 200 mol/m^3 V_a | volume of acid | 5×10^-5 m^3 [OH^-] | base concentration | 100 mol/m^3 | : V_b = (200 mol/m^3×5×10^-5 m^3)/(100 mol/m^3) Separate the numerical part, (200×5×10^-5)/100, from the unit part, (mol/m^3 m^3)/(mol/m^3) = m^3: V_b = (200×5×10^-5)/100 m^3 Evaluate (200×5×10^-5)/100: V_b = 1×10^-4 m^3 Convert 1×10^-4 m^3 into mL (milliliters) using the following: 1 m^3 = 1×10^6 mL: Answer: | | V_b = 100 mL
Titration plots
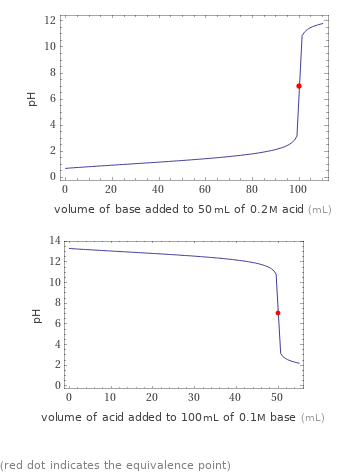
Titration plots
Units
