Input interpretation
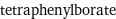
tetraphenylborate
Basic properties
(C3=CC=CC=C3)C4=CC=CC=C4 InChI identifier | InChI=1/C24H20B/c1-5-13-21(14-6-1)25(22-15-7-2-8-16-22, 23-17-9-3-10-18-23)24-19-11-4-12-20-24/h1-20H/q-1 InChI key | SVHQOIWIRUVWII-UHFFFAOYSA-N](../image_source/7b75a2d54d009b06f97b3b3dc28d5dbf.png)
molar mass | 319.2 g/mol formula | (C_24H_20B)^- empirical formula | C_24B_H_20 SMILES identifier | C1=CC=C(C=C1)[B-](C2=CC=CC=C2)(C3=CC=CC=C3)C4=CC=CC=C4 InChI identifier | InChI=1/C24H20B/c1-5-13-21(14-6-1)25(22-15-7-2-8-16-22, 23-17-9-3-10-18-23)24-19-11-4-12-20-24/h1-20H/q-1 InChI key | SVHQOIWIRUVWII-UHFFFAOYSA-N
Lewis structure
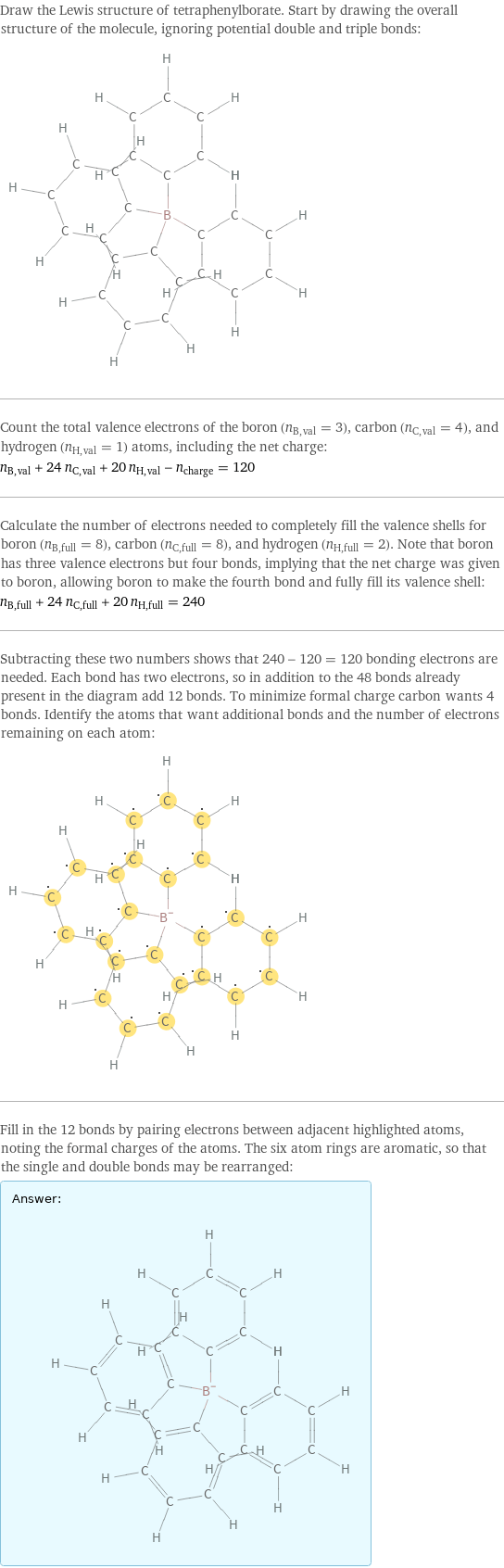
Draw the Lewis structure of tetraphenylborate. Start by drawing the overall structure of the molecule, ignoring potential double and triple bonds: Count the total valence electrons of the boron (n_B, val = 3), carbon (n_C, val = 4), and hydrogen (n_H, val = 1) atoms, including the net charge: n_B, val + 24 n_C, val + 20 n_H, val - n_charge = 120 Calculate the number of electrons needed to completely fill the valence shells for boron (n_B, full = 8), carbon (n_C, full = 8), and hydrogen (n_H, full = 2). Note that boron has three valence electrons but four bonds, implying that the net charge was given to boron, allowing boron to make the fourth bond and fully fill its valence shell: n_B, full + 24 n_C, full + 20 n_H, full = 240 Subtracting these two numbers shows that 240 - 120 = 120 bonding electrons are needed. Each bond has two electrons, so in addition to the 48 bonds already present in the diagram add 12 bonds. To minimize formal charge carbon wants 4 bonds. Identify the atoms that want additional bonds and the number of electrons remaining on each atom: Fill in the 12 bonds by pairing electrons between adjacent highlighted atoms, noting the formal charges of the atoms. The six atom rings are aromatic, so that the single and double bonds may be rearranged: Answer: | |
Quantitative molecular descriptors
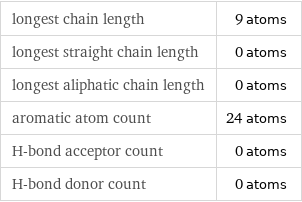
longest chain length | 9 atoms longest straight chain length | 0 atoms longest aliphatic chain length | 0 atoms aromatic atom count | 24 atoms H-bond acceptor count | 0 atoms H-bond donor count | 0 atoms
Elemental composition
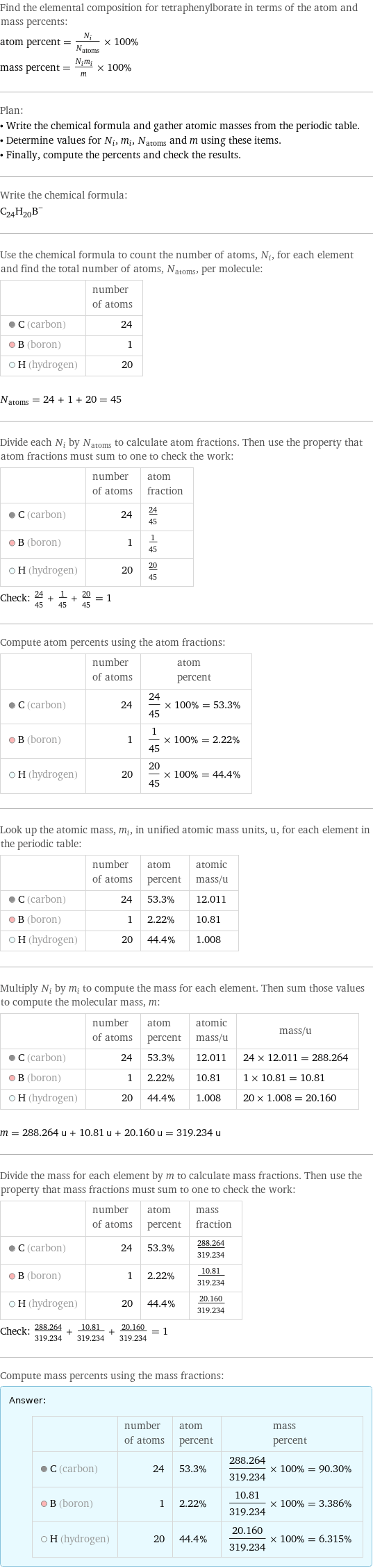
Find the elemental composition for tetraphenylborate in terms of the atom and mass percents: atom percent = N_i/N_atoms × 100% mass percent = (N_im_i)/m × 100% Plan: • Write the chemical formula and gather atomic masses from the periodic table. • Determine values for N_i, m_i, N_atoms and m using these items. • Finally, compute the percents and check the results. Write the chemical formula: (C_24H_20B)^- Use the chemical formula to count the number of atoms, N_i, for each element and find the total number of atoms, N_atoms, per molecule: | number of atoms C (carbon) | 24 B (boron) | 1 H (hydrogen) | 20 N_atoms = 24 + 1 + 20 = 45 Divide each N_i by N_atoms to calculate atom fractions. Then use the property that atom fractions must sum to one to check the work: | number of atoms | atom fraction C (carbon) | 24 | 24/45 B (boron) | 1 | 1/45 H (hydrogen) | 20 | 20/45 Check: 24/45 + 1/45 + 20/45 = 1 Compute atom percents using the atom fractions: | number of atoms | atom percent C (carbon) | 24 | 24/45 × 100% = 53.3% B (boron) | 1 | 1/45 × 100% = 2.22% H (hydrogen) | 20 | 20/45 × 100% = 44.4% Look up the atomic mass, m_i, in unified atomic mass units, u, for each element in the periodic table: | number of atoms | atom percent | atomic mass/u C (carbon) | 24 | 53.3% | 12.011 B (boron) | 1 | 2.22% | 10.81 H (hydrogen) | 20 | 44.4% | 1.008 Multiply N_i by m_i to compute the mass for each element. Then sum those values to compute the molecular mass, m: | number of atoms | atom percent | atomic mass/u | mass/u C (carbon) | 24 | 53.3% | 12.011 | 24 × 12.011 = 288.264 B (boron) | 1 | 2.22% | 10.81 | 1 × 10.81 = 10.81 H (hydrogen) | 20 | 44.4% | 1.008 | 20 × 1.008 = 20.160 m = 288.264 u + 10.81 u + 20.160 u = 319.234 u Divide the mass for each element by m to calculate mass fractions. Then use the property that mass fractions must sum to one to check the work: | number of atoms | atom percent | mass fraction C (carbon) | 24 | 53.3% | 288.264/319.234 B (boron) | 1 | 2.22% | 10.81/319.234 H (hydrogen) | 20 | 44.4% | 20.160/319.234 Check: 288.264/319.234 + 10.81/319.234 + 20.160/319.234 = 1 Compute mass percents using the mass fractions: Answer: | | | number of atoms | atom percent | mass percent C (carbon) | 24 | 53.3% | 288.264/319.234 × 100% = 90.30% B (boron) | 1 | 2.22% | 10.81/319.234 × 100% = 3.386% H (hydrogen) | 20 | 44.4% | 20.160/319.234 × 100% = 6.315%
Elemental oxidation states
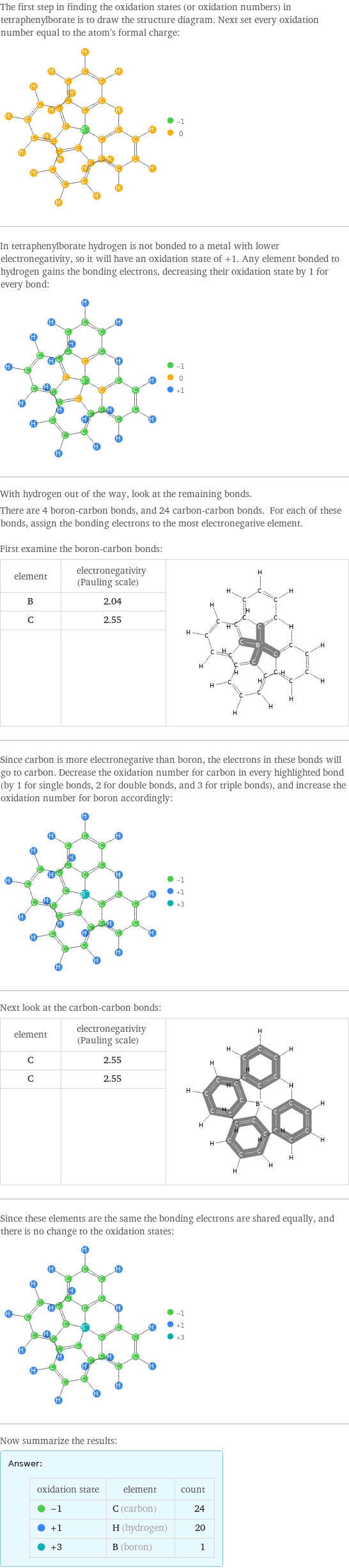
The first step in finding the oxidation states (or oxidation numbers) in tetraphenylborate is to draw the structure diagram. Next set every oxidation number equal to the atom's formal charge: In tetraphenylborate hydrogen is not bonded to a metal with lower electronegativity, so it will have an oxidation state of +1. Any element bonded to hydrogen gains the bonding electrons, decreasing their oxidation state by 1 for every bond: With hydrogen out of the way, look at the remaining bonds. There are 4 boron-carbon bonds, and 24 carbon-carbon bonds. For each of these bonds, assign the bonding electrons to the most electronegative element. First examine the boron-carbon bonds: element | electronegativity (Pauling scale) | B | 2.04 | C | 2.55 | | | Since carbon is more electronegative than boron, the electrons in these bonds will go to carbon. Decrease the oxidation number for carbon in every highlighted bond (by 1 for single bonds, 2 for double bonds, and 3 for triple bonds), and increase the oxidation number for boron accordingly: Next look at the carbon-carbon bonds: element | electronegativity (Pauling scale) | C | 2.55 | C | 2.55 | | | Since these elements are the same the bonding electrons are shared equally, and there is no change to the oxidation states: Now summarize the results: Answer: | | oxidation state | element | count -1 | C (carbon) | 24 +1 | H (hydrogen) | 20 +3 | B (boron) | 1
Orbital hybridization

First draw the structure diagram for tetraphenylborate, and for every non-hydrogen atom, count the σ-bonds. Note that double and triple bonds consist of one σ-bond together with one or two π-bonds: For tetraphenylborate there are no lone pairs, so the steric number is given by the σ-bond count. Consult the following chart to determine the hybridization from the steric number: steric number | hybridization 2 | sp 3 | sp^2 4 | sp^3 5 | dsp^3 6 | d^2sp^3 7 | d^3sp^3 Now assign the hybridization for each atom: Answer: | |
Topological indices

vertex count | 45 edge count | 48 Schultz index | 21036 Wiener index | 5184 Hosoya index | 8.556×10^8 Balaban index | 2.201