Input interpretation

hydrostatic pressure
Equation

P = ρ g h | P | pressure ρ | fluid density h | height g | standard acceleration due to gravity on the surface of the earth (≈ 9.807 m/s^2)
Units

Input values
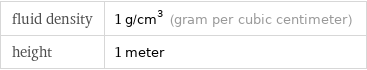
fluid density | 1 g/cm^3 (gram per cubic centimeter) height | 1 meter
Results

pressure | 9.807 kPa (kilopascals) = 1.422 psi (pounds-force per square inch) = 204.8 lbf/ft^2 (pounds-force per square foot) = 9807 Pa (pascals) = 0.09678 atm (atmospheres) = 98.07 mbar (millibars) = 73.56 mmHg (millimeters of mercury) = 100 cm WC (centimeters of water column)
Possible intermediate steps

Calculate the pressure using the following information: known variables | | ρ | fluid density | 1 g/cm^3 h | height | 1 m Convert known variables into appropriate units using the following: 1 g/cm^3 = 1×10^6 g/m^3: known variables | | ρ | fluid density | 1×10^6 g/m^3 h | height | 1 m The relevant equation that relates pressure (P), fluid density (ρ), and height (h) is: P = ρ g h Substitute known variables and constants into the equation: known variables | | ρ | fluid density | 1×10^6 g/m^3 h | height | 1 m constant | | g | standard acceleration due to gravity on the surface of the earth | 196133/20000 m/s^2 | : P = 1×10^6 g/m^3×9.8067 m/s^2×1 m Separate the numerical part, 1×10^6×9.8067×1, from the unit part, g/m^3×m/s^2 m = g/(m s^2): P = 1×10^6×9.8067×1 g/(m s^2) Evaluate 1×10^6×9.8067×1: P = 9.8067×10^6 g/(m s^2) Convert 9.8067×10^6 g/(m s^2) into kPa (kilopascals) using the following: 1 g/(m s^2) = 1×10^-6 kPa: Answer: | | P = 9.807 kPa
Schematic
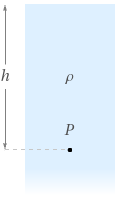
Schematic