name of 1, 1-bis(3, 4-dimethylphenyl)ethane

1, 1-bis(3, 4-dimethylphenyl)ethane

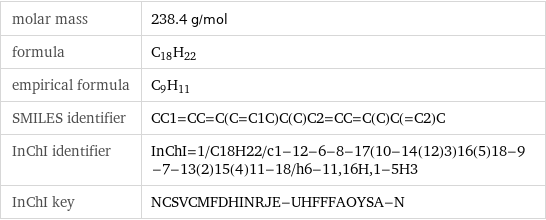
molar mass | 238.4 g/mol formula | C_18H_22 empirical formula | C_9H_11 SMILES identifier | CC1=CC=C(C=C1C)C(C)C2=CC=C(C)C(=C2)C InChI identifier | InChI=1/C18H22/c1-12-6-8-17(10-14(12)3)16(5)18-9-7-13(2)15(4)11-18/h6-11, 16H, 1-5H3 InChI key | NCSVCMFDHINRJE-UHFFFAOYSA-N

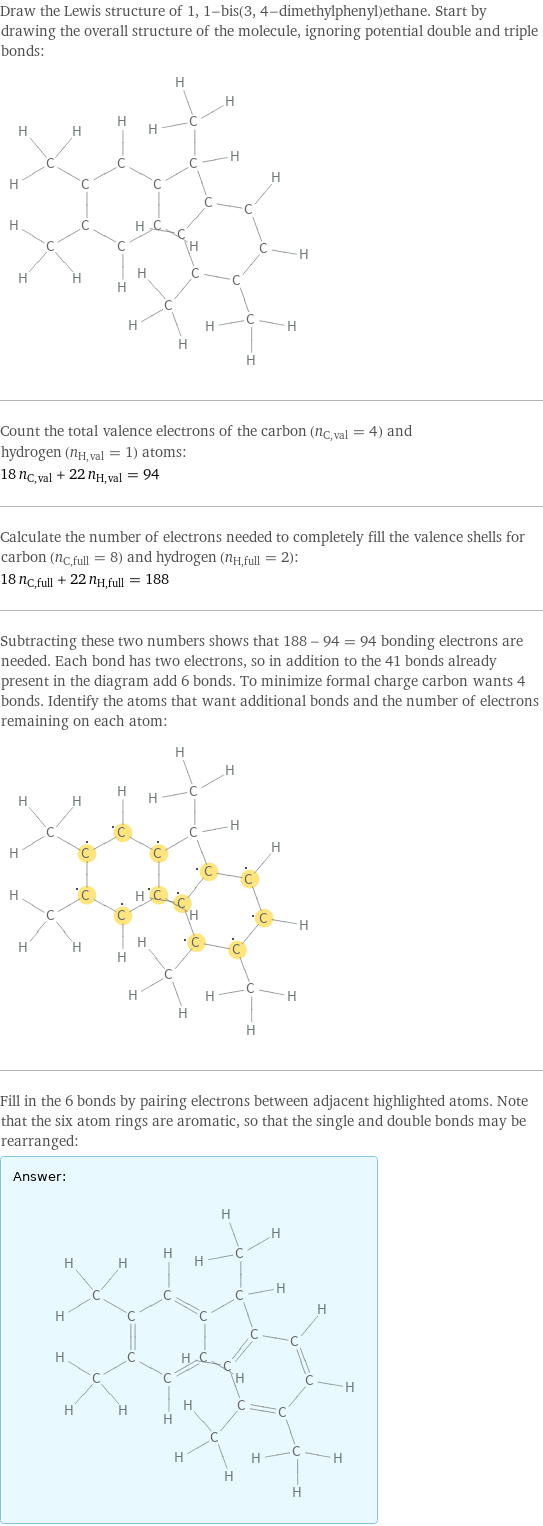
Draw the Lewis structure of 1, 1-bis(3, 4-dimethylphenyl)ethane. Start by drawing the overall structure of the molecule, ignoring potential double and triple bonds: Count the total valence electrons of the carbon (n_C, val = 4) and hydrogen (n_H, val = 1) atoms: 18 n_C, val + 22 n_H, val = 94 Calculate the number of electrons needed to completely fill the valence shells for carbon (n_C, full = 8) and hydrogen (n_H, full = 2): 18 n_C, full + 22 n_H, full = 188 Subtracting these two numbers shows that 188 - 94 = 94 bonding electrons are needed. Each bond has two electrons, so in addition to the 41 bonds already present in the diagram add 6 bonds. To minimize formal charge carbon wants 4 bonds. Identify the atoms that want additional bonds and the number of electrons remaining on each atom: Fill in the 6 bonds by pairing electrons between adjacent highlighted atoms. Note that the six atom rings are aromatic, so that the single and double bonds may be rearranged: Answer: | |

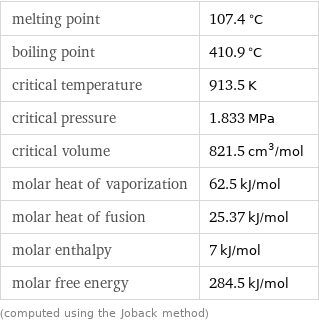
melting point | 107.4 °C boiling point | 410.9 °C critical temperature | 913.5 K critical pressure | 1.833 MPa critical volume | 821.5 cm^3/mol molar heat of vaporization | 62.5 kJ/mol molar heat of fusion | 25.37 kJ/mol molar enthalpy | 7 kJ/mol molar free energy | 284.5 kJ/mol (computed using the Joback method)

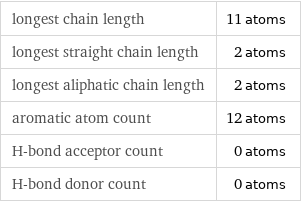
longest chain length | 11 atoms longest straight chain length | 2 atoms longest aliphatic chain length | 2 atoms aromatic atom count | 12 atoms H-bond acceptor count | 0 atoms H-bond donor count | 0 atoms

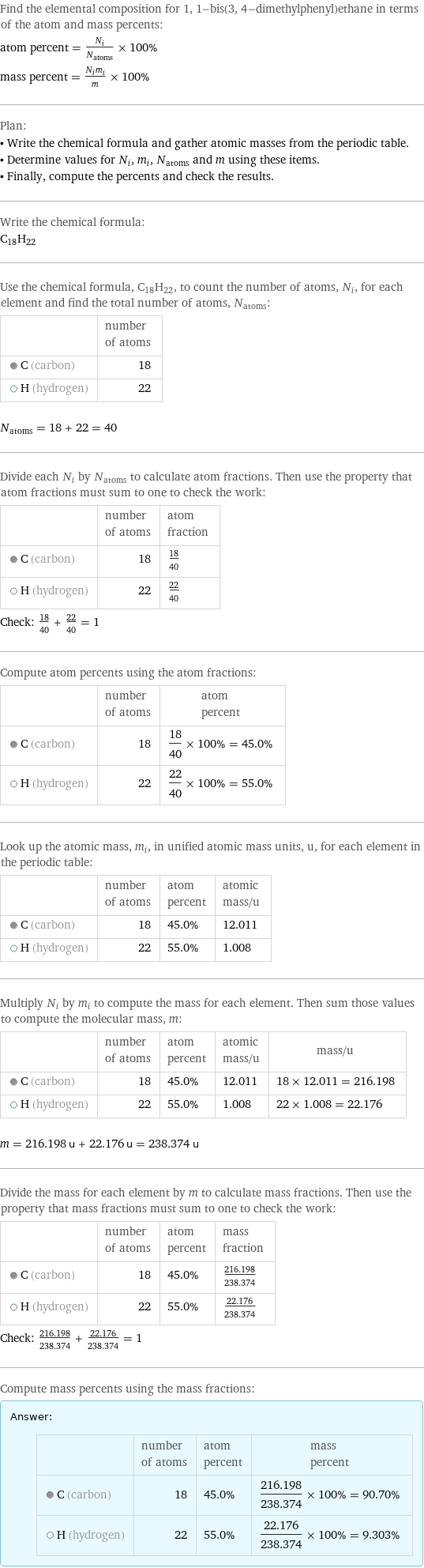
Find the elemental composition for 1, 1-bis(3, 4-dimethylphenyl)ethane in terms of the atom and mass percents: atom percent = N_i/N_atoms × 100% mass percent = (N_im_i)/m × 100% Plan: • Write the chemical formula and gather atomic masses from the periodic table. • Determine values for N_i, m_i, N_atoms and m using these items. • Finally, compute the percents and check the results. Write the chemical formula: C_18H_22 Use the chemical formula, C_18H_22, to count the number of atoms, N_i, for each element and find the total number of atoms, N_atoms: | number of atoms C (carbon) | 18 H (hydrogen) | 22 N_atoms = 18 + 22 = 40 Divide each N_i by N_atoms to calculate atom fractions. Then use the property that atom fractions must sum to one to check the work: | number of atoms | atom fraction C (carbon) | 18 | 18/40 H (hydrogen) | 22 | 22/40 Check: 18/40 + 22/40 = 1 Compute atom percents using the atom fractions: | number of atoms | atom percent C (carbon) | 18 | 18/40 × 100% = 45.0% H (hydrogen) | 22 | 22/40 × 100% = 55.0% Look up the atomic mass, m_i, in unified atomic mass units, u, for each element in the periodic table: | number of atoms | atom percent | atomic mass/u C (carbon) | 18 | 45.0% | 12.011 H (hydrogen) | 22 | 55.0% | 1.008 Multiply N_i by m_i to compute the mass for each element. Then sum those values to compute the molecular mass, m: | number of atoms | atom percent | atomic mass/u | mass/u C (carbon) | 18 | 45.0% | 12.011 | 18 × 12.011 = 216.198 H (hydrogen) | 22 | 55.0% | 1.008 | 22 × 1.008 = 22.176 m = 216.198 u + 22.176 u = 238.374 u Divide the mass for each element by m to calculate mass fractions. Then use the property that mass fractions must sum to one to check the work: | number of atoms | atom percent | mass fraction C (carbon) | 18 | 45.0% | 216.198/238.374 H (hydrogen) | 22 | 55.0% | 22.176/238.374 Check: 216.198/238.374 + 22.176/238.374 = 1 Compute mass percents using the mass fractions: Answer: | | | number of atoms | atom percent | mass percent C (carbon) | 18 | 45.0% | 216.198/238.374 × 100% = 90.70% H (hydrogen) | 22 | 55.0% | 22.176/238.374 × 100% = 9.303%

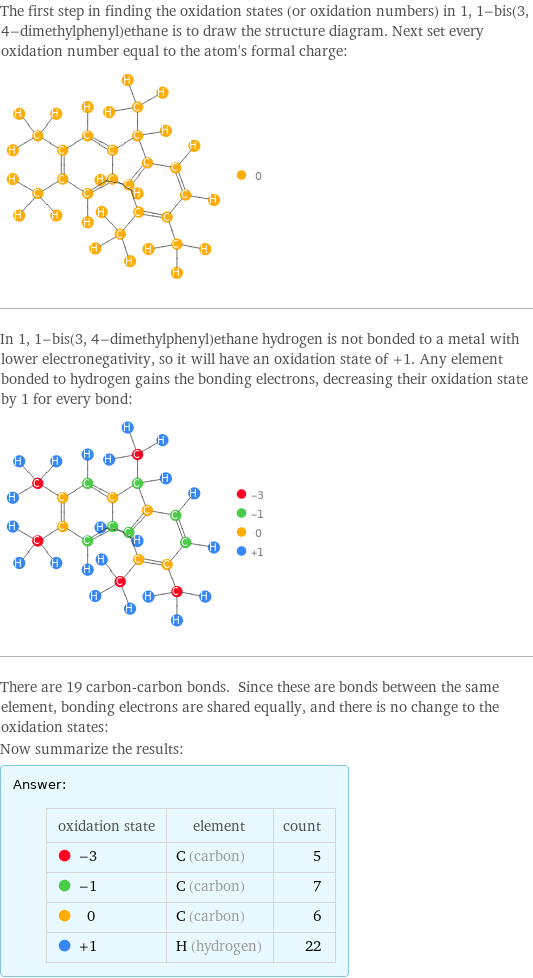
The first step in finding the oxidation states (or oxidation numbers) in 1, 1-bis(3, 4-dimethylphenyl)ethane is to draw the structure diagram. Next set every oxidation number equal to the atom's formal charge: In 1, 1-bis(3, 4-dimethylphenyl)ethane hydrogen is not bonded to a metal with lower electronegativity, so it will have an oxidation state of +1. Any element bonded to hydrogen gains the bonding electrons, decreasing their oxidation state by 1 for every bond: There are 19 carbon-carbon bonds. Since these are bonds between the same element, bonding electrons are shared equally, and there is no change to the oxidation states: Now summarize the results: Answer: | | oxidation state | element | count -3 | C (carbon) | 5 -1 | C (carbon) | 7 0 | C (carbon) | 6 +1 | H (hydrogen) | 22

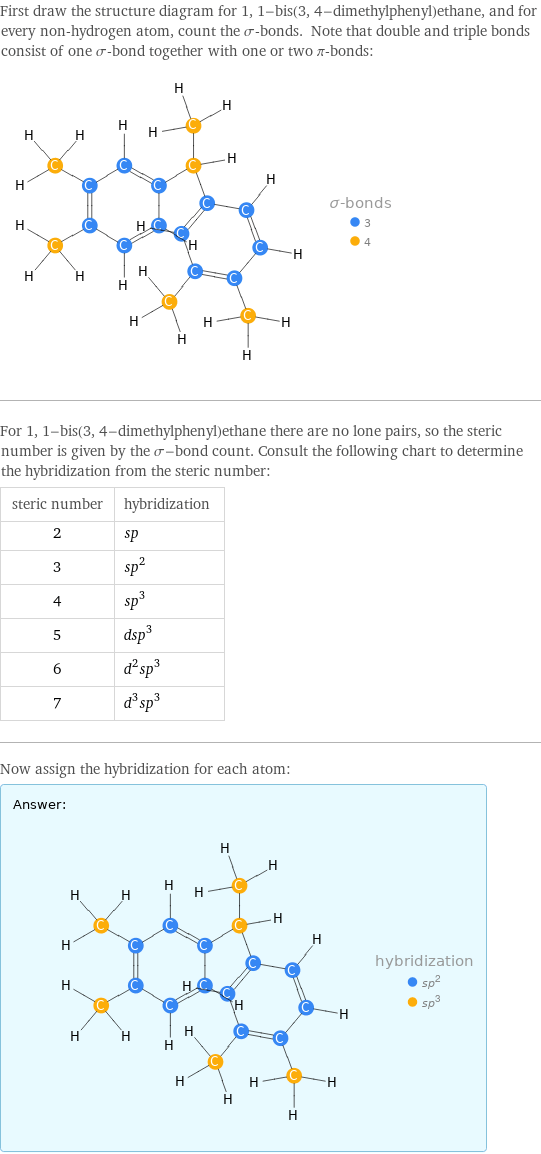
First draw the structure diagram for 1, 1-bis(3, 4-dimethylphenyl)ethane, and for every non-hydrogen atom, count the σ-bonds. Note that double and triple bonds consist of one σ-bond together with one or two π-bonds: For 1, 1-bis(3, 4-dimethylphenyl)ethane there are no lone pairs, so the steric number is given by the σ-bond count. Consult the following chart to determine the hybridization from the steric number: steric number | hybridization 2 | sp 3 | sp^2 4 | sp^3 5 | dsp^3 6 | d^2sp^3 7 | d^3sp^3 Now assign the hybridization for each atom: Answer: | |

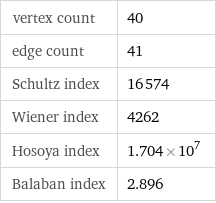
vertex count | 40 edge count | 41 Schultz index | 16574 Wiener index | 4262 Hosoya index | 1.704×10^7 Balaban index | 2.896