Input interpretation

Abbe number (for helium)
Equation
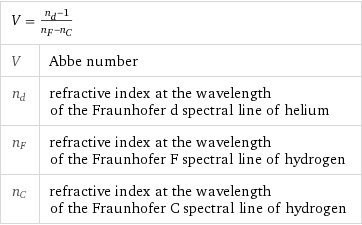
V = (n_d - 1)/(n_F - n_C) | V | Abbe number n_d | refractive index at the wavelength of the Fraunhofer d spectral line of helium n_F | refractive index at the wavelength of the Fraunhofer F spectral line of hydrogen n_C | refractive index at the wavelength of the Fraunhofer C spectral line of hydrogen
Input values

refractive index at the wavelength of the Fraunhofer d spectral line of helium | 1.3 refractive index at the wavelength of the Fraunhofer F spectral line of hydrogen | 1.4 refractive index at the wavelength of the Fraunhofer C spectral line of hydrogen | 1.35
Results

Abbe number | 6
Possible intermediate steps
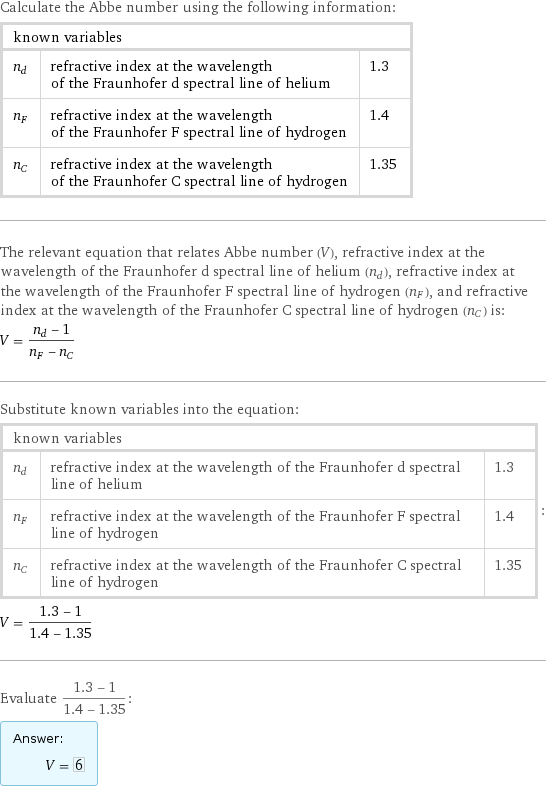
Calculate the Abbe number using the following information: known variables | | n_d | refractive index at the wavelength of the Fraunhofer d spectral line of helium | 1.3 n_F | refractive index at the wavelength of the Fraunhofer F spectral line of hydrogen | 1.4 n_C | refractive index at the wavelength of the Fraunhofer C spectral line of hydrogen | 1.35 The relevant equation that relates Abbe number (V), refractive index at the wavelength of the Fraunhofer d spectral line of helium (n_d), refractive index at the wavelength of the Fraunhofer F spectral line of hydrogen (n_F), and refractive index at the wavelength of the Fraunhofer C spectral line of hydrogen (n_C) is: V = (n_d - 1)/(n_F - n_C) Substitute known variables into the equation: known variables | | n_d | refractive index at the wavelength of the Fraunhofer d spectral line of helium | 1.3 n_F | refractive index at the wavelength of the Fraunhofer F spectral line of hydrogen | 1.4 n_C | refractive index at the wavelength of the Fraunhofer C spectral line of hydrogen | 1.35 | : V = (1.3 - 1)/(1.4 - 1.35) Evaluate (1.3 - 1)/(1.4 - 1.35): Answer: | | V = 6