Input interpretation

aeschynite-(Nd) (mineral) | crystal system | woodallite (mineral) | crystal system
Result
orthorhombic | trigonal
Basic properties

| orthorhombic | trigonal crystal families | orthorhombic | hexagonal required symmetries | 3 2-fold rotation axes or 1 2-fold rotation axis and 2 mirror planes | 1 3-fold rotation axis Bravais lattices | 4 | 2 point groups | 3 | 5 space groups | 59 | 25
Lattice properties
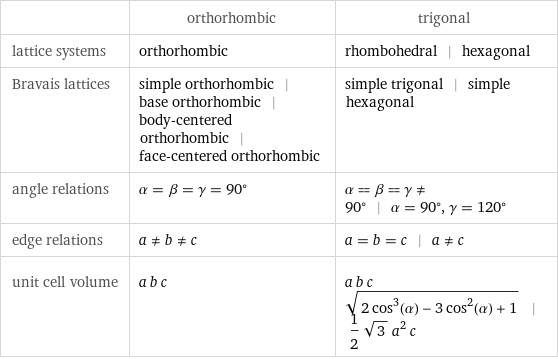
| orthorhombic | trigonal lattice systems | orthorhombic | rhombohedral | hexagonal Bravais lattices | simple orthorhombic | base orthorhombic | body-centered orthorhombic | face-centered orthorhombic | simple trigonal | simple hexagonal angle relations | α = β = γ = 90° | α = β = γ!=90° | α = 90°, γ = 120° edge relations | a!=b!=c | a = b = c | a!=c unit cell volume | a b c | a b c sqrt(2 cos^3(α) - 3 cos^2(α) + 1) | 1/2 sqrt(3) a^2 c
Corresponding symmetry groups

| orthorhombic | trigonal crystal class | orthorhombic sphenoidal | orthorhombic pyramidal | orthorhombic bipyramidal | trigonal pyramidal | rhombohedral | trigonal trapezoidal | ditrigonal pyramidal | ditrigonal scalahedral Schönflies point groups | {D_2, C_2v, D_2h} | {C_3, S_6, D_3, C_3v, D_3d} Hermann-Mauguin point groups | 222 | mm2 | mmm | 3 | 3^_ | 32 | 3m | 3^_m IUCr space group number | 16 | 17 | 18 | 19 | 20 | 21 | 22 | 23 | 24 | 25 | ... (total: 59) | 143 | 144 | 145 | 146 | 147 | 148 | 149 | 150 | 151 | 152 | ... (total: 25) Hermann-Mauguin space groups | P222 | P222_1 | P2_12_12 | P2_12_12_1 | C222_1 | C222 | F222 | I222 | I2_12_12_1 | Pmm2 | ... (total: 59) | P3 | P3_1 | P3_2 | R3 | P3^_ | R3^_ | P312 | P321 | P3_112 | P3_121 | ... (total: 25)