Input interpretation
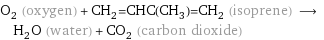
O_2 (oxygen) + CH_2=CHC(CH_3)=CH_2 (isoprene) ⟶ H_2O (water) + CO_2 (carbon dioxide)
Balanced equation

Balance the chemical equation algebraically: O_2 + CH_2=CHC(CH_3)=CH_2 ⟶ H_2O + CO_2 Add stoichiometric coefficients, c_i, to the reactants and products: c_1 O_2 + c_2 CH_2=CHC(CH_3)=CH_2 ⟶ c_3 H_2O + c_4 CO_2 Set the number of atoms in the reactants equal to the number of atoms in the products for O, C and H: O: | 2 c_1 = c_3 + 2 c_4 C: | 5 c_2 = c_4 H: | 8 c_2 = 2 c_3 Since the coefficients are relative quantities and underdetermined, choose a coefficient to set arbitrarily. To keep the coefficients small, the arbitrary value is ordinarily one. For instance, set c_2 = 1 and solve the system of equations for the remaining coefficients: c_1 = 7 c_2 = 1 c_3 = 4 c_4 = 5 Substitute the coefficients into the chemical reaction to obtain the balanced equation: Answer: | | 7 O_2 + CH_2=CHC(CH_3)=CH_2 ⟶ 4 H_2O + 5 CO_2
Structures
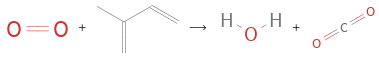
+ ⟶ +
Names

oxygen + isoprene ⟶ water + carbon dioxide
Equilibrium constant
![K_c = ([H2O]^4 [CO2]^5)/([O2]^7 [CH2=CHC(CH3)=CH2])](../image_source/cfa62cb35c08034085947a70150230dc.png)
K_c = ([H2O]^4 [CO2]^5)/([O2]^7 [CH2=CHC(CH3)=CH2])
Rate of reaction
![rate = -1/7 (Δ[O2])/(Δt) = -(Δ[CH2=CHC(CH3)=CH2])/(Δt) = 1/4 (Δ[H2O])/(Δt) = 1/5 (Δ[CO2])/(Δt) (assuming constant volume and no accumulation of intermediates or side products)](../image_source/38de0d04ea0520a14d21122aac9f7c38.png)
rate = -1/7 (Δ[O2])/(Δt) = -(Δ[CH2=CHC(CH3)=CH2])/(Δt) = 1/4 (Δ[H2O])/(Δt) = 1/5 (Δ[CO2])/(Δt) (assuming constant volume and no accumulation of intermediates or side products)
Chemical names and formulas

| oxygen | isoprene | water | carbon dioxide formula | O_2 | CH_2=CHC(CH_3)=CH_2 | H_2O | CO_2 Hill formula | O_2 | C_5H_8 | H_2O | CO_2 name | oxygen | isoprene | water | carbon dioxide IUPAC name | molecular oxygen | isoprene | water | carbon dioxide