Input interpretation

single-slit diffraction
Equation

sin(θ_R) = λ/d | θ_R | Rayleigh criterion angle λ | wavelength d | slit width (valid in far-field limit (Fraunhofer diffraction))
Input values
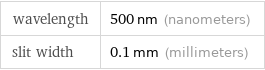
wavelength | 500 nm (nanometers) slit width | 0.1 mm (millimeters)
Results

Rayleigh criterion angle | 0.2865° (degrees) = 5 mrad (milliradians) = 0.005 radians = 17 arc minutes 11.33 arc seconds = 17.19' (arc minutes)
Possible intermediate steps
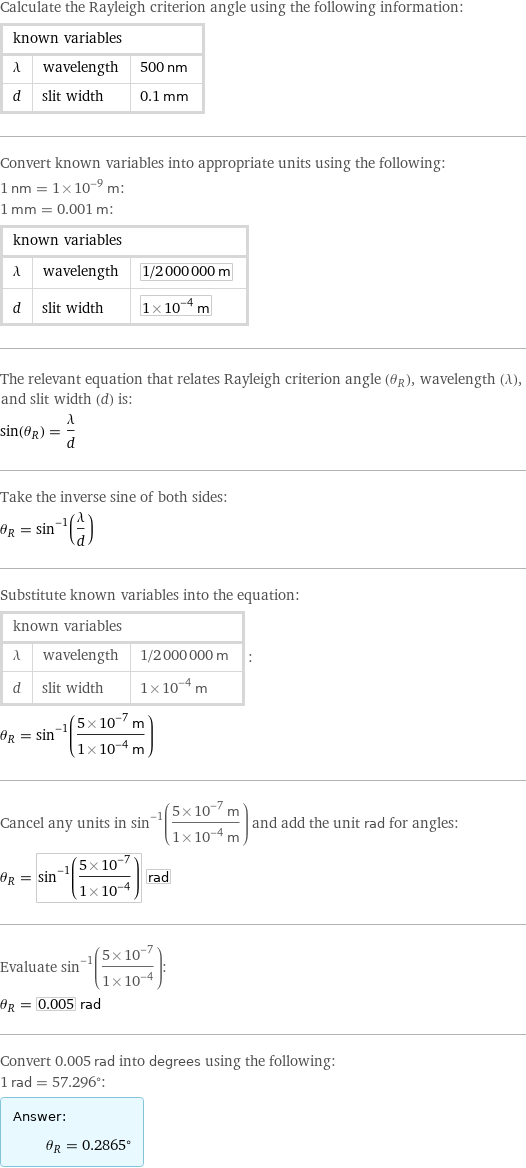
Calculate the Rayleigh criterion angle using the following information: known variables | | λ | wavelength | 500 nm d | slit width | 0.1 mm Convert known variables into appropriate units using the following: 1 nm = 1×10^-9 m: 1 mm = 0.001 m: known variables | | λ | wavelength | 1/2000000 m d | slit width | 1×10^-4 m The relevant equation that relates Rayleigh criterion angle (θ_R), wavelength (λ), and slit width (d) is: sin(θ_R) = λ/d Take the inverse sine of both sides: θ_R = sin^(-1)(λ/d) Substitute known variables into the equation: known variables | | λ | wavelength | 1/2000000 m d | slit width | 1×10^-4 m | : θ_R = sin^(-1)((5×10^-7 m)/(1×10^-4 m)) Cancel any units in sin^(-1)((5×10^-7 m)/(1×10^-4 m)) and add the unit rad for angles: θ_R = sin^(-1)((5×10^-7)/(1×10^-4)) rad Evaluate sin^(-1)((5×10^-7)/(1×10^-4)): θ_R = 0.005 rad Convert 0.005 rad into degrees using the following: 1 rad = 57.296°: Answer: | | θ_R = 0.2865°
Diffraction pattern
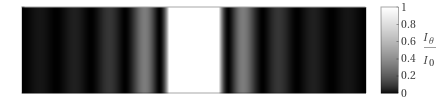
Diffraction pattern
Normalized transmitted intensity vs. diffraction angle
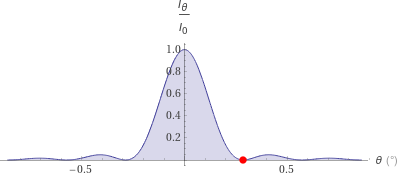
Normalized transmitted intensity vs. diffraction angle
Zeros of transmitted intensity as a function of diffraction angle
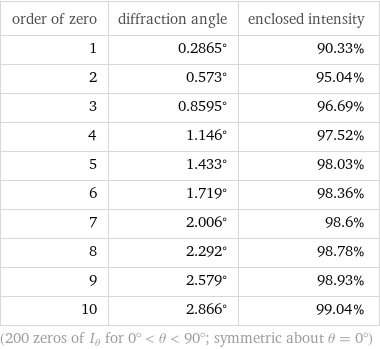
order of zero | diffraction angle | enclosed intensity 1 | 0.2865° | 90.33% 2 | 0.573° | 95.04% 3 | 0.8595° | 96.69% 4 | 1.146° | 97.52% 5 | 1.433° | 98.03% 6 | 1.719° | 98.36% 7 | 2.006° | 98.6% 8 | 2.292° | 98.78% 9 | 2.579° | 98.93% 10 | 2.866° | 99.04% (200 zeros of I_θ for 0° < θ < 90°; symmetric about θ = 0°)