Input interpretation

strontium cyanide dihydrate | molar mass
Result
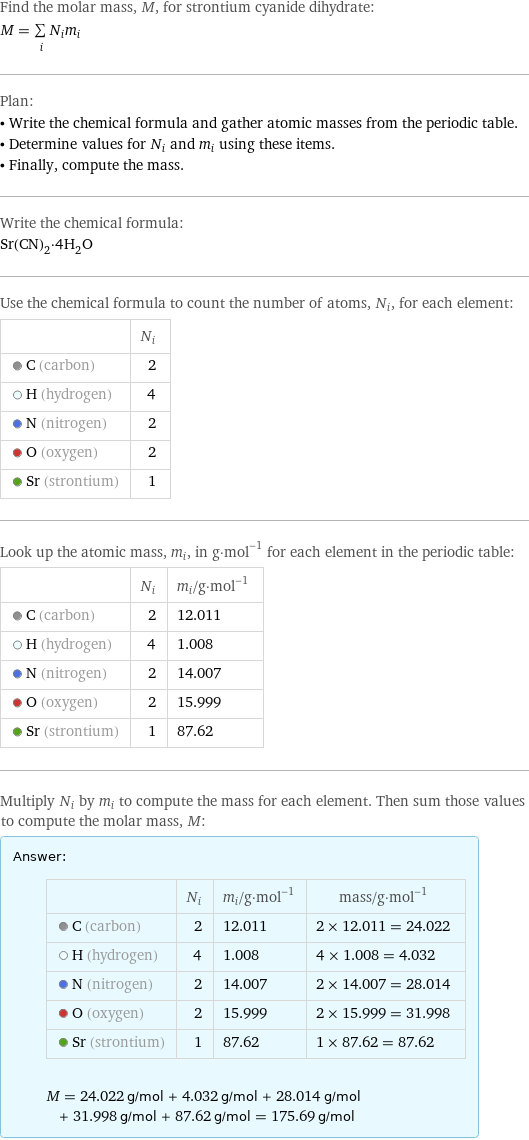
Find the molar mass, M, for strontium cyanide dihydrate: M = sum _iN_im_i Plan: • Write the chemical formula and gather atomic masses from the periodic table. • Determine values for N_i and m_i using these items. • Finally, compute the mass. Write the chemical formula: Sr(CN)_2·4H_2O Use the chemical formula to count the number of atoms, N_i, for each element: | N_i C (carbon) | 2 H (hydrogen) | 4 N (nitrogen) | 2 O (oxygen) | 2 Sr (strontium) | 1 Look up the atomic mass, m_i, in g·mol^(-1) for each element in the periodic table: | N_i | m_i/g·mol^(-1) C (carbon) | 2 | 12.011 H (hydrogen) | 4 | 1.008 N (nitrogen) | 2 | 14.007 O (oxygen) | 2 | 15.999 Sr (strontium) | 1 | 87.62 Multiply N_i by m_i to compute the mass for each element. Then sum those values to compute the molar mass, M: Answer: | | | N_i | m_i/g·mol^(-1) | mass/g·mol^(-1) C (carbon) | 2 | 12.011 | 2 × 12.011 = 24.022 H (hydrogen) | 4 | 1.008 | 4 × 1.008 = 4.032 N (nitrogen) | 2 | 14.007 | 2 × 14.007 = 28.014 O (oxygen) | 2 | 15.999 | 2 × 15.999 = 31.998 Sr (strontium) | 1 | 87.62 | 1 × 87.62 = 87.62 M = 24.022 g/mol + 4.032 g/mol + 28.014 g/mol + 31.998 g/mol + 87.62 g/mol = 175.69 g/mol
Unit conversion
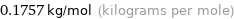
0.1757 kg/mol (kilograms per mole)
Comparisons
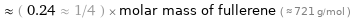
≈ ( 0.24 ≈ 1/4 ) × molar mass of fullerene ( ≈ 721 g/mol )
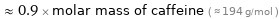
≈ 0.9 × molar mass of caffeine ( ≈ 194 g/mol )
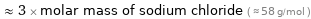
≈ 3 × molar mass of sodium chloride ( ≈ 58 g/mol )
Corresponding quantities
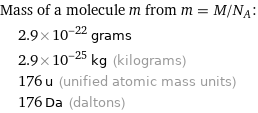
Mass of a molecule m from m = M/N_A: | 2.9×10^-22 grams | 2.9×10^-25 kg (kilograms) | 176 u (unified atomic mass units) | 176 Da (daltons)
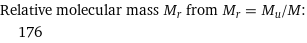
Relative molecular mass M_r from M_r = M_u/M: | 176