Input interpretation
constructive thin film interference
Equation
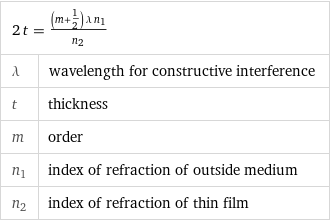
2 t = ((m + 1/2) λ n_1)/n_2 | λ | wavelength for constructive interference t | thickness m | order n_1 | index of refraction of outside medium n_2 | index of refraction of thin film
Input values
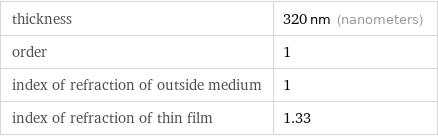
thickness | 320 nm (nanometers) order | 1 index of refraction of outside medium | 1 index of refraction of thin film | 1.33
Results

wavelength for constructive interference | 567.5 nm (nanometers) = 2.234×10^-5 inches = 0.5675 µm (micrometers) = 5.675×10^-7 meters
Possible intermediate steps
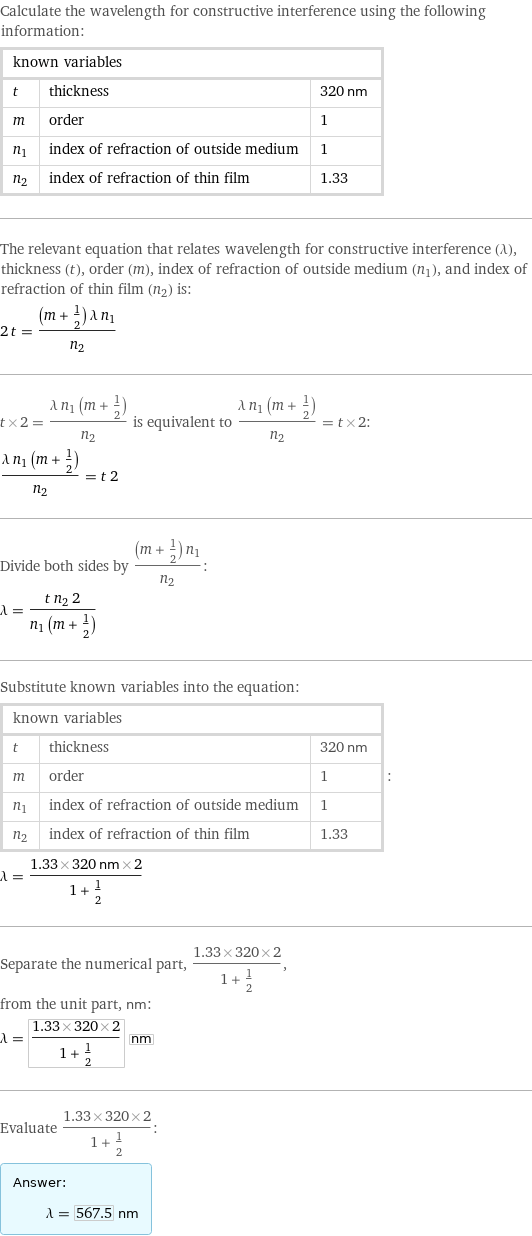
Calculate the wavelength for constructive interference using the following information: known variables | | t | thickness | 320 nm m | order | 1 n_1 | index of refraction of outside medium | 1 n_2 | index of refraction of thin film | 1.33 The relevant equation that relates wavelength for constructive interference (λ), thickness (t), order (m), index of refraction of outside medium (n_1), and index of refraction of thin film (n_2) is: 2 t = ((m + 1/2) λ n_1)/n_2 t×2 = (λ n_1 (m + 1/2))/n_2 is equivalent to (λ n_1 (m + 1/2))/n_2 = t×2: (λ n_1 (m + 1/2))/n_2 = t 2 Divide both sides by ((m + 1/2) n_1)/n_2: λ = (t n_2 2)/(n_1 (m + 1/2)) Substitute known variables into the equation: known variables | | t | thickness | 320 nm m | order | 1 n_1 | index of refraction of outside medium | 1 n_2 | index of refraction of thin film | 1.33 | : λ = (1.33×320 nm×2)/(1 + 1/2) Separate the numerical part, (1.33×320×2)/(1 + 1/2), from the unit part, nm: λ = (1.33×320×2)/(1 + 1/2) nm Evaluate (1.33×320×2)/(1 + 1/2): Answer: | | λ = 567.5 nm