Input interpretation
catheter flow rate
Equation
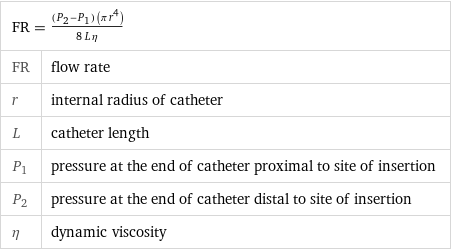
FR = ((P_2 - P_1) (π r^4))/(8 L η) | FR | flow rate r | internal radius of catheter L | catheter length P_1 | pressure at the end of catheter proximal to site of insertion P_2 | pressure at the end of catheter distal to site of insertion η | dynamic viscosity
Input values

internal radius of catheter | 0.3 mm (millimeters) catheter length | 15 cm (centimeters) pressure at the end of catheter proximal to site of insertion | 1900 Pa (pascals) pressure at the end of catheter distal to site of insertion | 3100 Pa (pascals) dynamic viscosity | 0.00682 poise
Results

flow rate | 0.03731 mL/s (milliliters per second) = 0.03548 gal/h (gallons per hour) = 2.239 mL/min (milliliters per minute) = 0.002239 L/min (liters per minute) = 134.3 mL/h (milliliters per hour) = 3.224 L/day (liters per day) = 2.239 cm^3/min (cubic centimeters per minute)
Possible intermediate steps

Calculate the flow rate using the following information: known variables | | r | internal radius of catheter | 0.3 mm L | catheter length | 15 cm P_1 | pressure at the end of catheter proximal to site of insertion | 1900 Pa P_2 | pressure at the end of catheter distal to site of insertion | 3100 Pa η | dynamic viscosity | 0.00682 P Convert known variables into appropriate units using the following: 1 mm = 0.001 m: 1 cm = 0.01 m: 1 Pa = 1000 g/(m s^2): 1 Pa = 1000 g/(m s^2): 1 P = 100 g/(m s): known variables | | r | internal radius of catheter | 3×10^-4 m L | catheter length | 3/20 m P_1 | pressure at the end of catheter proximal to site of insertion | 1.9×10^6 g/(m s^2) P_2 | pressure at the end of catheter distal to site of insertion | 3.1×10^6 g/(m s^2) η | dynamic viscosity | 0.682 g/(m s) The relevant equation that relates flow rate (FR), internal radius of catheter (r), catheter length (L), pressure at the end of catheter proximal to site of insertion (P_1), pressure at the end of catheter distal to site of insertion (P_2), and dynamic viscosity (η) is: FR = ((P_2 - P_1) (π r^4))/(8 L η) Substitute known variables and constants into the equation: known variables | | r | internal radius of catheter | 3×10^-4 m L | catheter length | 3/20 m P_1 | pressure at the end of catheter proximal to site of insertion | 1.9×10^6 g/(m s^2) P_2 | pressure at the end of catheter distal to site of insertion | 3.1×10^6 g/(m s^2) η | dynamic viscosity | 0.682 g/(m s) constant | | π | pi | 3.141593 | : FR = ((3.1×10^6 g/(m s^2) - 1.9×10^6 g/(m s^2)) (3.141593 (3×10^-4 m)^4))/(8×0.15 m×0.682 g/(m s)) Separate the numerical part, ((3.1×10^6 - 1.9×10^6) (3.141593 (3×10^-4)^4))/(8×0.15×0.682), from the unit part, ((g/(m s^2) + g/(m s^2)) m^4)/(m×g/(m s)) = m^3/s: FR = ((3.1×10^6 - 1.9×10^6) (3.141593 (3×10^-4)^4))/(8×0.15×0.682) m^3/s Evaluate ((3.1×10^6 - 1.9×10^6) (3.141593 (3×10^-4)^4))/(8×0.15×0.682): FR = 3.73122×10^-8 m^3/s Convert 3.73122×10^-8 m^3/s into mL/s (milliliters per second) using the following: 1 m^3/s = 1×10^6 mL/s: Answer: | | FR = 0.03731 mL/s