Input interpretation

radiocarbon years
Equation

t(BP) = -t_Libby log(2, N/N_0) | t(BP) | radiocarbon years before present N | remaining number of radiocarbon atoms N_0 | initial number of radiocarbon atoms t_Libby | Libby half-life for radioactive decay of carbon-14 (≈ 5568 years)
Input values
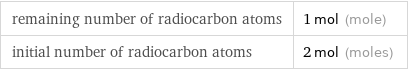
remaining number of radiocarbon atoms | 1 mol (mole) initial number of radiocarbon atoms | 2 mol (moles)
Result
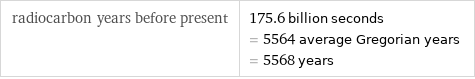
radiocarbon years before present | 175.6 billion seconds = 5564 average Gregorian years = 5568 years