Input interpretation
Vickers hardness
Equation

HV = (F 2 sin((136 °)/2))/d^2 | HV | Vickers hardness F | applied force d | indentation diagonal average length
Input values

applied force | 30 kgf (kilograms-force) indentation diagonal average length | 0.5 mm (millimeters)
Results
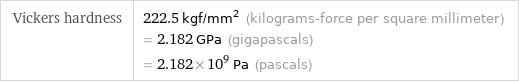
Vickers hardness | 222.5 kgf/mm^2 (kilograms-force per square millimeter) = 2.182 GPa (gigapascals) = 2.182×10^9 Pa (pascals)
Possible intermediate steps

Calculate the Vickers hardness using the following information: known variables | | F | applied force | 30 kgf d | indentation diagonal average length | 0.5 mm Convert known variables into appropriate units using the following: 1 kgf = 9806.6 g m/s^2: 1 mm = 0.001 m: known variables | | F | applied force | 588399/2 g m/s^2 d | indentation diagonal average length | 5×10^-4 m The relevant equation that relates Vickers hardness (HV), applied force (F), and indentation diagonal average length (d) is: HV = (2 (F sin((136 °)/2)))/d^2 Substitute known variables and constants into the equation: known variables | | F | applied force | 588399/2 g m/s^2 d | indentation diagonal average length | 5×10^-4 m constant | | ° | Degree | 0.0174533 | : HV = (2 (294200 g m/s^2 sin((136×0.0174533)/2)))/(5×10^-4 m)^2 Separate the numerical part, (2 (294200 sin((136×0.0174533)/2)))/(5×10^-4)^2, from the unit part, (g m/s^2)/(m^2) = g/(m s^2): HV = (2 (294200 sin((136×0.0174533)/2)))/(5×10^-4)^2 g/(m s^2) Evaluate (2 (294200 sin((136×0.0174533)/2)))/(5×10^-4)^2: HV = 2.1822×10^12 g/(m s^2) Convert 2.1822×10^12 g/(m s^2) into kgf/mm^2 (kilograms-force per square millimeter) using the following: 1 g/(m s^2) = 1.0197×10^-10 kgf/mm^2: Answer: | | HV = 222.5 kgf/mm^2
Approximate system conversions
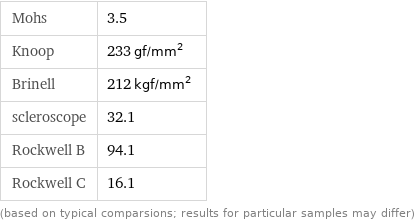
Mohs | 3.5 Knoop | 233 gf/mm^2 Brinell | 212 kgf/mm^2 scleroscope | 32.1 Rockwell B | 94.1 Rockwell C | 16.1 (based on typical comparsions; results for particular samples may differ)
Units

Hardness comparisons
harder than calcite (Mohs 3)
softer than fluorite (Mohs 4)